Next: 7.2.3 Existential elimination Up: 7.2 Rules about quantifiers Previous: 7.2.1 What's that Contents
If we see a proof of its existence, we can say that a property is true for some element:
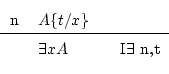
That ![]() is a substitution (maybe read ``
is a substitution (maybe read ``![]() over
over ![]() '' and is done by changing
'' and is done by changing ![]() to
to ![]() ).
).
This rule says that if we see ![]() , where
, where ![]() is any element, we
can say that
is any element, we
can say that ![]() , because we know that when
, because we know that when ![]() is
is ![]() then the formula is true.
then the formula is true.